Method for min./max. thrust
In this method used by Coyne and Belier engineers (Francq, 1993), at a given elevation (e.g., concrete lift joint), the static equilibrium of a corner of the backfill is considered according to Coulomb's analysis and its weight is divided between a force on the dam (R) and a force on the underlying backfill (F). The smallest possible embankment action is sought to guarantee the stability of the dam (with normal or exceptional reservoir levels). At the same time, the greatest possible thrust that can be exerted on an empty dam is also sought to ensure that this force does not cause the upstream overturning of the dam.

Figure 215 Method for max./min. thrust. – minimum thrust
In the first case (Figure 215) the smallest possible thrust force is calculated assuming a Rankine equilibrium for the backfill. The failure occurs along a plane at an angle of (450 + ϕ / 2) to the horizontal, where ϕ is the angle of internal friction of the embankment. An angle of ϕ / 2 is assumed between the action (R) of the backfill on the dam and the normal to the face (the assumption of zero friction on the downstream face would benefit the stability). As for the directions of the internal interaction forces F, according to Coulomb's analysis, they are inclined by ϕ from the failure plane. These interaction forces pass through the lower third of the failure plane.
The actual forces developed by the backfill probably exceed the minimum value, but this difference is nevertheless conservative. With these assumptions, only a minimum force and its associated moment are considered for the stability of the retrofitted dam. In reality, the backfill thrust force will certainly be reached or exceeded.
For a rockfill, the value of ϕ = 450 is recommended by Francq (1993). Note that this 'design value' does not have to be reached by the actual friction of the placed riprap. This value may seem high, but any decrease in the angle of friction below the recommended value of 450 increases the thrust force and raises it horizontally, which further stabilizes the gravity dam. On the other hand, it is not desirable to place riprap with a friction angle greater than 450 that would provide little benefit.
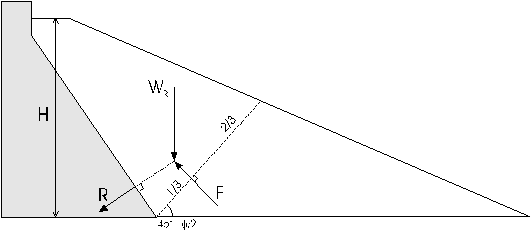
Figure 116 Method for max./min. thrust. – maximum thrust
With an empty reservoir, this is the maximum thrust force sought (Figure 216). It is assumed that there is no internal friction in the backfill (ϕ = 0) and the procedure is the same as described above. Zero friction is also assumed on the failure plane, which is the most pessimistic assumption, thus raising the thrust force developed by the backfill wedge. The wedge cut by a failure plane at (450 + ϕ/2) = 450 is approximately the maximum wedge likely to rest on the downstream face of the dam.